Introduction
Regular arrangement of atoms in molecules and extended solids is very common. In crystalline solids the atoms are arranged in repeating three-dimensional arrays or lattices. Information regarding atomic bond distances and angles is fundamental to understanding the chemical and physical properties of materials. Since atomic dimensions are of the order of angstroms (10-10m), unraveling the relative atomic positions of a solid requires a physical technique that operates on a similar spatial scale, Diffraction experiments involving X-ray, electron and neutron sources have therefore played a very crucial role in unraveling these structures. The significance of these experiments in science and engineering courses has been recognized. However, the cost of the equipment and the hazards associated with these experiments have made them very difficult to be integrated and included in a teaching laboratory setting.

The present set-up overcomes these limitations. An increase in scale by thousands from short  wavelengths of X-rays to the long wavelengths of visible light, and by hundreds of thousands from an array of atoms in a crystal or an extended solid to an array of dots, allows us to replicate the basic features of a structural determination experiment in a teaching laboratory.

In place of Bragg diffraction whose results are to be simulated, we use Fraunhofer diffraction. Visible laser light passes through an array of scattering centers (dots) on a 35 mm slide. The diffraction pattern is viewed at what is effectively infinite distance (a meter or so). This arrangement is capable of illustrating many of the essential features of the standard X-ray experiment. Mathematically, the equations for Fraunhofer and Bragg diffraction have a similar functional dependence on the interatomic distance, wavelength and the scattering angle. The symmetry of the diffraction pattern is same as the symmetry of the lattice causing the diffraction.

The central piece of the set-up is a slide (transparency) with eight (A-H) different arrangements of scattering centers (dots) on different portions of the slide (Figure 3). The examples presented here serve to illustrate how the spacings, symmetries, spot intensities and systematic absences in a diffraction pattern are related to the lattice from which it is derived. Although these are two—dimensional lattices, they mimic what would be observed for diffraction from particular three-dimensional structures that are viewed in projection perpendicular to a face.

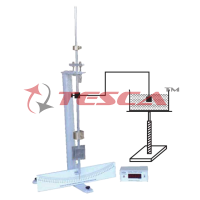
Setup
A mains operated constant current laser light, mounted on a stand is put at one end of an optical bench. The slide is mounted on another stand (Figure 1) which is kept at about 10-20 cm away from the laser such that the laser light falls normally on the slide. The slide on this stand can be moved sideways and vertically so that the laser light falls on different portions of the slide. The diffraction pattern is observed on a perspex screen (Figure 2) fixed normal to the laser light at the other end of the optical bench. The whole set-up is put in a darkened room where the experiment is performed.